Majorana fermions in the Kitaev quantum spin system α-RuCl₃

Abstract

Geometrical constraints to the electronic degrees of freedom within condensed-matter systems often give rise to topological quantum states of matter such as fractional quantum Hall states, topological insulators, and Weyl semimetals^1,2,3. In magnetism, theoretical studies predict an entangled magnetic quantum state with topological ordering and fractionalized spin excitations, the quantum spin liquid⁴. In particular, the so-called Kitaev spin model⁵, consisting of a network of spins on a honeycomb lattice, is predicted to host Majorana fermions as its excitations. By means of a combination of specific heat measurements and inelastic neutron scattering experiments, we demonstrate the emergence of Majorana fermions in single crystals of α-RuCl₃, an experimental realization of the Kitaev spin lattice. The specific heat data unveils a two-stage release of magnetic entropy that is characteristic of localized and itinerant Majorana fermions. The neutron scattering results corroborate this picture by revealing quasielastic excitations at low energies around the Brillouin zone centre and an hour-glass-like magnetic continuum at high energies. Our results confirm the presence of Majorana fermions in the Kitaev quantum spin liquid and provide an opportunity to build a unified conceptual framework for investigating fractionalized excitations in condensed matter^1,6,7,8.

Main

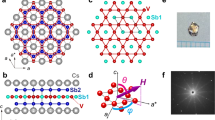
Quantum spin liquids (QSLs) are an unconventional electronic phase of matter characterized by an absence of magnetic long-range order down to zero temperature. They are typically predicted to occur in geometrically frustrated magnets such as triangular, kagome, and pyrochlore lattices⁴, and typically display a macroscopic degeneracy that stabilizes a topologically ordered ground state. The Kitaev QSL state arises as an exact solution of the ideal two-dimensional (2D) honeycomb lattice with bond-directional Ising-type interactions (H = J_K^γS_i^γS_j^γ; γ = x, y, z) on the three distinct links (Fig. 1a) by expressing the spin excitations in terms of non-interacting Majorana fermions^5,9. The elementary excitations of a Kitaev QSL are localized and itinerant Majorana fermions⁵, which are associates with static Z₂-fluxes and propagating quasiparticles (Fig. 1b). These two types of excitation have ramifications for the observable physics and potential technological applications of QSL in quantum computers^{10,11,12,13,14}.

Figure 1: Kitaev bonding geometry and cartoon of emergent Majorana fermions.

a, Local Ru³⁺ (J_eff = 1/2; 4d⁵) hexagon structure formed by the edge-shared RuCl₆ octahedra, in the layered honeycomb material α-RuCl₃. Two 94° Ru–Cl–Ru superexchange paths lead to the Kitaev interactions J_K^γS_i^γS_j^γ between two magnetic spins on adjacent i and j sites, and the three different links denoted with γ (= x, y, z) contribute isotropic J_K in the rhombohedral crystal structure. The Pauli spin operators can be represented by σ_i^γ = ib_i^γc_i in terms of localized (b_i^γ) and itinerant (c_i) Majorana fermions in an extended Hilbert space. The plaquette operator W_p, a product of the bond operators u_ij^γ = ib_i^γb_j^γ around the hexagon, results in the Z₂ gauge fluxes of 0 (eigenvalue w_p = + 1) and π (w_p = −1) in the Kitaev lattice. b, (Left) In very low-temperature T < T_L, the Z₂-fluxes are almost frozen in the quantum spin liquid ground state (yellow hexagon) and only low-energy itinerant Majorana fermions (black balls) are thermally activated. As temperature increases, the spin excitations are thermally fractionalized into itinerant and localized (cyan ovals) Majorana fermions. (Middle) In the intermediate temperature T_L < T < T_H, the π-fluxes (red hexagons) become populated by the thermal energy and the itinerant Majorana fermions on the vertices move in a coherent manner. As temperature crosses over T_H, the nearest-neighbour spin–spin correlation is diminished. (Right) Finally, in high temperature T ≫ T_H, the system becomes a conventional paramagnet.

As candidates for realizing a QSL, honeycomb iridates A₂IrO₃ (A = Li, Na) with a spin–orbit coupled J_eff = 1/2 Ir⁴⁺ (5d⁵) state¹⁵ have been intensively studied. This is due to the orbital state forming the three orthogonal bonds required for the bond-directional exchange interactions in the geometry¹⁶. The iridates, however, cannot avoid monoclinic distortions with anisotropic Ir–Ir bonds disturbing the exchange frustration away from the ideal values, and their magnetism is apparently dominated by antiferromagnetic (AFM) ordering^17,18.

A promising candidate for the Kitaev model system is the van der Waals ruthenate α-RuCl₃ with J_eff = 1/2 Ru³⁺ (4d⁵) ions^19,20. There is a growing body of evidence that α-RuCl₃ hosts predominantly Ising-like Kitaev interactions and that the ground state could be proximate to the QSL state^21,22. Most crystallographic studies reported the presence of the monoclinic distortions^23,24, resulting in considerable contribution of Heisenberg and asymmetric exchange interactions^25,26. However, these distortions are probably due to stacking faults of the RuCl₃ layers, and even lead to multiple magnetic transitions²⁴. Recently, significant advances in the synthesis of high-quality α-RuCl₃ crystals have been achieved. These crystals are almost free from stacking faults and have a rhombohedral () phase, while preserving the Ising-type AFM state below 6.5 K due to non-vanishing inter-layer couplings²⁷. Importantly, this high-symmetry structure renders isotropic Kitaev interactions (J_K = J_K^x = J_K^y = J_K^z) with a 94° Ru–Cl–Ru bond angle maximizing the Kitaev interaction, and the Heisenberg contribution becomes minimal²⁶. Furthermore, recent methodological progress in the quantum Monte Carlo (QMC) method and cluster dynamic mean-field theory (CDMFT) for thermally excited quantum states provides a route to identify Majorana fermions emerging from the QSL ground state^12,13,14. It is predicted that thermally fluctuating quantum spins are successively fractionalized into itinerant and localized Majorana fermions at crossover temperatures T_L (low-T) and T_H (high-T), respectively. At very low-temperature (T < T_L), Z₂-fluxes are mostly frozen in the topologically ordered zero-temperature QSL state and the thermal energy excites only low-energy itinerant Majorana fermions (see Fig. 1b). On increasing the temperature across T_L, the fluxes fluctuate to activate localized Majorana fermions (Kitaev paramagnet). Upon further heating, itinerant Majorana fermions are additionally activated and the spin–spin correlation fades out across T_H. Finally, the system ends in a conventional paramagnetic phase well above T_H.

Figure 2 displays the thermodynamic signatures in the magnetic susceptibility χ(T), magnetic specific heat C_M and entropy S_M for fractionalized spin excitations. The static χ(T) of α-RuCl₃ deviates from the Curie–Weiss curve below 140 K, indicating the onset of short-range spin correlations (Fig. 2a). The anomalies in χ(T) and C_M at T_N = 6.5 K represent the onset of zigzag-type AFM order (Fig. 2a, b). C_M is obtained by subtracting the lattice contribution from the total specific heat (C_P) as described in the Supplementary Information. Besides the sharp anomaly at T_N, C_M exhibits two broad maxima, one near T_N and the other around T_H ≈ 100 K, although the low-T maximum feature is obscured by the AFM anomaly. As predicted in theory^12,13, the high- and low-T structures can be ascribed to the thermal excitations of itinerant and localized Majorana fermions, respectively. It is worth noting that C_M follows a linear T-dependence in the intermediate range T_N < T < T_H, reflecting metallic-like behaviour of itinerant Majorana fermions (inset of Fig. 2b).

Figure 2: Thermodynamic signatures of spin fractionalization.

a, Temperature-dependent static magnetic susceptibility of α-RuCl₃ plotted in a semi-log scale for H ∥ ab. The susceptibility deviates from the Curie–Weiss behaviour (solid red line) below T = 140 K. The low-temperature kink at T_N = 6.5 K indicates a zigzag-type AFM order. b, Magnetic specific heat C_M obtained by subtracting the lattice contribution in a semi-log scale (see Supplementary Information). Besides the AFM peak at T_N, the broad bumps in T_N ≲ T ≲ 50 K and around T = T_H ≃ 100 K (vertical bar) are associated with excitations of localized and itinerant Majorana fermions, respectively. C_M exhibits a T-linear dependence in the intermediate temperature 50 K ≲ T ≲ T_H, as shown in the inset, reflecting metal-like density of states of the itinerant Majorana fermions. The spike at 165 K is due to a structural phase transition. c, Magnetic entropy change, integrated C_M, in the temperature range 2 K < T < 200 K. The horizontal solid lines represent the expected total entropy change Rln2 and its half value (R/2)ln2. The solid red line is a sum of two phenomenological function fits based on the theoretical simulation, indicating that the entropy release is decomposed into two fermionic components (yellow and green shadings), as described in the Supplementary Information.

Rather firm evidence is provided by the two-stage release of the entropy gain S_M(T) = ∫ C_M/TdT (Fig. 2c). The obtained S_M at T = 200 K is 5.13 J mol⁻¹ K⁻¹, which corresponds to about 90 % of the ideal value Rln2 (R: ideal gas constant) of the spin-1/2 system. Upon cooling, nearly half of the entropy is released stepwise with the plateau-like behaviour at 0.46Rln2, signifying two maxima of C_M. Indeed, S_M(T) above T_N agrees well with the simulated sum (red line) of two phenomenological Schottky-like functions with about an equal weight (ρ_H = 0.92, ρ_L = 1.08, T_H ≃ 101 K, and T_L ≃ 22 K), which involve itinerant and localized Majorana fermions in the QMC simulation (see Supplementary Information). Considering the predicted temperature ratio T_L/T_H ≈ 0.03 in the isotropic Kitaev model, T_L would be somewhat lower than T_N if the AFM order were absent. S_M involving AFM order below T_N was estimated to be 1.09 J mol⁻¹ K⁻¹, about 20% of the total entropy Rln2 (40% of 1/2Rln2) (ref. 27), indicating that the entropy held by the AFM order is partially released and roughly 3/5ths of the frozen Z₂-flux is maintained just above T_N.

The microscopic and dynamic properties of the Majorana fermions can be visualized by the thermally fractionalized spin excitations obtained from the INS measurements. Figure 3a shows the neutron scattering function S_tot(Q, ω) as a function of momentum transfer Q and energy transfer ω measured at T = 10 K above T_N along the X–K–Γ–M–Y direction. S_tot(Q, ω) at sufficiently low T can be approximated as the magnetic scattering function S_mag(Q, ω) although weak phonon features are still observable as marked with black stars in the figure (see Supplementary Information). S_tot(Q, ω) displays an hour-glass shape spectrum centred at the Γ-pointextending to about 20 meV with strong low-energy excitations around the Γ-point and high-energy Y-shaped excitations. Similar features are reproduced in the simulated spectra of the isotropic Kitaev model with a FM Kitaev interaction J_K = −16.5 meV by using the CDMFT + continuous-time QMC method¹⁴ (see Fig. 3b). It is worth noting that the spectral centre would move to the M-point for an AFM J_K (>0) (ref. 14). The low-energy feature represents the quasielastic responses associated with the flux excitations, and the Y-shaped Q-ω dependence in the high-energy region reflects the dispersive itinerant Majorana fermions extending to ω ∼ |J_K| (refs 11,14). Both features are also clearly observable in the constant-energy cuts S_tot(Q), which also agree well with the theoretical calculations (Fig. 3c). According to the simulation, the excitation energy of the itinerant MF at the K- and M-points corresponds to Kitaev J_K. S_tot(Q) data (Fig. 3d) are again compared with the simulated values (Fig. 3e) in 2D reciprocal space (Fig. 3f). The overall features are well reproduced by the simulations, except the hexagram-shaped Q-dependence of the low-energy S_tot(Q) (ω ≲ 6 meV), indicating that the key character of the Majorana fermions is rather robust. The hexagram-shaped Q-dependence is considered to be induced by the second nearest-neighbour Kitaev interactions²⁸ and/or symmetric anisotropy exchange interactions^29,30 involving direct Ru–Ru electron hopping, both of which are not considered in the pure Kitaev model. These interactions are weak, but become important at low energies and temperatures.

Figure 3: Magnetic excitation spectra of α-RuCl₃ compared with the theoretical calculations on a ferromagnetic Kitaev model.

a, Neutron scattering function S_tot (Q, ω) at T = 10 K along the high symmetric line X–K–Γ–M–Y through Brillouin zone. The data were collected with an incoming neutron energy of E_i = 31 meV (MERLIN). The black stars mark phonons (see Supplementary Information). b, Calculated magnetic scattering function S_mag(Q, ω) for a ferromagnetic Kitaev model at T = 0.06|J_K|. c, Constant-energy cuts integrated over the energy ranges [3, 5], [5, 7], [8, 10], [11, 13], [14, 16], and [17, 19] meV along the X–K–Γ and Γ–M–Y directions (left y-axis). The dashed lines guide vertical offsets. The solid lines present the theoretical calculations of the pure Kitaev model (right y-axis). Error bars represent one standard deviation. d, Constant-energy cuts in the (hk)-plane integrated over the energy ranges [1.5, 2.5], [4, 6] (LET, E_i = 10 meV), [9, 12] (LET, E_i = 22 meV), and [16, 19] meV (MERLIN, E_i = 31 meV). e, Constant-energy cuts of the theoretical S_mag(Q) in the Kitaev model for comparison. f, The reciprocal honeycomb lattice in the space group. The X–K–Γ and Γ–M–Y directions are presented with the red arrows. The white regions in a,d mark the lack of detector coverage. The colour bars in a,d are represented in units of mbarn sr⁻¹ meV⁻¹ per Ru. The calculations presented in b,e are dimensionless, with the scale given by the colour bar.

Figure 4a, b presents the thermal evolution of the experimental and simulated S_mag(Q, ω) (see Methods), respectively. At T = 16 K, the hour-glass shape spectrum is maintained with minor reduction in the overall intensity. Upon heating up to T_H ∼ 100 K (Kitaev paramagnetic phase), the low-energy intensity involving localized Majorana fermions is significantly reduced while the high-energy intensity from itinerant Majorana fermions is almost maintained, although the dichotomic feature becomes smeared with increasing thermal fluctuations. Further heating across T_H causes the high-energy intensity to begin to decrease considerably. Well above T_H(T = 240 K), S_mag(Q, ω) exhibits only a featureless low background as in conventional paramagnets. The evolution of localized and itinerant Majorana fermions with temperature are visualized in the temperature–energy contour plots of S_mag around the Γ-point, as presented in Fig. 4c (experiment) and 4d (simulation). The low-energy excitations below ω ≈ 4 meV appear at T ≲ T_H while the high-energy excitations extend out to ω ∼ |J_K|. This is also evident from the S_mag(Γ, ω) plots in Fig. 4e, which are consistent with the simulations.

Figure 4: Evolution of the two Majorana fermion excitations.

a, Magnetic scattering function S_mag (Q, ω) at T = 16, 75, 125, and 240 K. The two data sets with an incoming neutron energy of E_i = 22 meV (upper panel) and 10 meV (down panel) are combined together. The white regions mark the lack of detector coverage. b, Calculated S_mag(Q, ω) at T = 0.09, 0.375, 0.69, and 1.32|J_K| with J_K = −16.5 meV for comparison with the experimental data. c,d, Comparison of contour plot of the experimental S_mag(Γ, ω) and the calculated S_mag(Γ, ω) in the temperature–energy plane. e, S_mag(Γ, ω) at T = 16 K (black circles), 75 K (yellow circles), 125 K (green circles), and 240 K (blue circles) as a function of energy. The calculated S_mag(Γ, ω) (the solid lines) are presented together for comparison. f,g, Temperature dependence of the integrated S_mag(Γ, ω) over the energy range ω₁ = [1.5, 3] meV (grey circles) and ω₂ = [8,14.5] meV (blue circles). Both energy ranges are marked with grey and blue areas in e, respectively. The cross symbols represent the calculated results of the integrated S_mag(Γ, ω), and the dashed lines represent the linear interpolations. The experimental S_mag(Γ, ω) in c,e–g are obtained by integrating the S_mag(Q, ω) within the area |H| ≤ 0.12 (reciprocal lattice unit) and |K| ≤ 0.2 in the HK-plane. The areas of diagonal stripes in c,d,f,g indicate the high-T crossover at T_H. The colour bars in a,c are represented in units of mbarn sr⁻¹ meV⁻¹ per Ru. The calculations presented in b,d are dimensionless, with the scale given by the colour bar. In e–g, measured and calculated S_mag refer to the left and right y-axes, respectively. Error bars represent one standard deviation.

The quantitative agreement between the experiment and the simulation is also excellent in the INS intensities for the low- and high-energy excitations in an overall temperature range, as shown in Fig. 4f, g, presenting the temperature dependences of the corresponding integrations ∫ S_mag(Γ, ω)dω. Meanwhile, one also notices that the experiment deviates somewhat from the simulation below ∼50 K only in the integration involving the low-energy excitations (Fig. 4f). This is probably due to the presence of the additional perturbing magnetic interactions in the real system, whose influence might be apparent in the low-energy scale to be detrimental to the low-energy flux excitations at low temperature. Those perturbing interactions contribute the hexagram-shaped Q-dependence in the low-energy S_mag(Q) (see Fig. 3d), which becomes isotropic above ∼50 K, as expected in the Kitaev model (see Supplementary Information).

Tracing the magnetic entropy and evolution of the spin excitations as a function of temperature, energy, and momentum, we provide strong evidence for thermal fractionalization to Majorana fermions of spin excitations. α-RuCl₃ is well described in the ferromagnetic Kitaev model and is proximate to the Kitaev QSL. The key features of the thermal fractionalization predicted in the pure Kitaev model are reproduced well in the thermodynamic and spectroscopic results, although AFM order is developed below T_N = 6.5 K and additional perturbing magnetic interactions deteriorate QSL behaviour, especially in the low-energy scale. When the temperature is higher than the energy scale related to the perturbing magnetic interactions, the two distinct Majorana fermions predicted in the Kitaev honeycomb model are unveiled. This finding lays a cornerstone for an in-depth understanding of emergent Majorana quasiparticles in condensed matter, and also possibly for future implementation in quantum computations.

Methods

Crystal growth.

High-quality single crystals of α-RuCl₃ and their isostructural counterpart ScCl₃ were grown by a vacuum sublimation method. A commercial RuCl₃ (ScCl₃) powder (Alfa Aesar) was thoroughly ground, and dehydrated in a quartz ampoule for a day. The ampoule was sealed in vacuum and placed in a temperature gradient furnace. The temperature of the RuCl₃ (ScCl₃) powder is set at 1,080 °C (900 °C). After dwelling for 5 h, the furnace is cooled to 650 °C (600 °C) at a rate of −2 °C per hour. We obtained α-RuCl₃ (ScCl₃) crystals black coloured (transparent) with shiny surfaces. Electron-dispersive X-ray measurements confirmed the stoichiometry of the Ru(Sc):Cl = 1:3 ratio for the crystals.

Magnetic susceptibility and specific heat measurement.

Magnetic susceptibility measurements were performed using a commercial superconducting quantum interference device (SQUID) (Quantum Design, model: MPMS-5XL). A single domain crystal (3 × 3 × 1 mm³, 20 mg) was chosen for the measurements under an external magnetic field parallel to the ab-plane. Specific heat C_P was measured by using a conventional calorimeter of the Quantum Design Physical Property Measurement System (model: PPMS DynaCool) in a temperature range of T = 1.8–300 K. The magnetic specific heat C_M of α-RuCl₃ was determined by subtracting the lattice contribution, which is supposed to be equivalent to the specific heat of the isostructural non-magnetic ScCl₃ with mass scaling (see Supplementary Information).

Inelastic neutron scattering.

Inelastic neutron scattering data were collected by using the time-of-flight spectrometers MERLIN (high intensity) and LET (high resolution) at the ISIS Spallation Neutron Source, the Rutherford Appleton Laboratory in the United Kingdom. Total 46 pieces (∼1.35 g) of α-RuCl₃ single crystals for MERLIN, and 153 pieces (∼5.1 g) for LET were prepared, and co-aligned with crystallographic c-axis surface normal on aluminium plates, resulting in a mosaic within 3° (Supplementary Fig. 1). The samples were mounted in a liquid helium cryostat for temperature control ranging from 1.5 K to 270 K. Due to the highly two-dimensional structure of α-RuCl₃, magnetic correlations between honeycomb layers are extremely weak and insensitive. Therefore, crystals are aligned with the c-axis parallel to the incident neutron beam, so that the area detector measures the energy spectrum over the 2D q-space of the hk-plane. To observe the intensity at the Γ-point (LET measurement), we rotated the crystal by 30 degrees to the incident beam direction, so that it filled the blank region of the beam mask.

Data were obtained with the incident neutron energy set to E_i = 5.66, 10, 22 (LET), and 31 meV (MERLIN). With incoherent neutron scattering intensity measured from a vanadium standard sample, all data were normalized and converted to the value of the neutron scattering function S_tot(Q, ω), which is proportional to the differential neutron cross-section (d²σ)/(dΩdE) and the ratio of the incident to the scattered neutron wavevector k_i/k_f (ref. 31),

Since S_tot(Q, ω) contains both the nuclear and magnetic scattering contributions, the magnetic scattering function S_mag(Q, ω)_T at temperature T in Fig. 4 is extracted from S_tot(Q, ω)_T after subtraction of the scaled ${\text{S}}_{tot}{(Q,\omega )}_{T_0=290\text{K}}$ with the Bose factor correction $n(T)/n(T_0)=(1-e^{-\hslash \omega /k_{\text{B}}{T}_0})/(1-e^{-\hslash \omega /k_{\text{B}}T})$, which represents the approximate phonon contribution in the experiment.

All of the data processes, including Bose factor correction and projection of the scattering function along appropriate directions, were performed using the HORACE software, which is published by ISIS³².

Calculation of the magnetic scattering function.

The calculation of S_mag(Q, ω)_T is performed by using the CDMFT + continuous-time QMC method as described in ref. 15. The Bose factor correction of the equation (2) is also applied to the simulation results for quantitative comparison with the experimental results in Fig. 4. All calculated results include the magnetic form factor of the Ru³⁺ ion, which is obtained by using the density functional theory method considering solid-state effects in α-RuCl₃, as described in the Supplementary Information.

Data availability.

The data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.