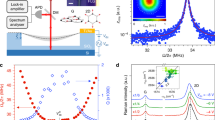
Abstract
Many of the applications of graphene rely on its uneven stiffness and high thermal conductivity, but the mechanical properties of graphene—and, in general, of all two-dimensional materials—are still not fully understood. Harmonic theory predicts a quadratic dispersion for the out-of-plane flexural acoustic vibrational mode, which leads to the unphysical result that long-wavelength in-plane acoustic modes decay before vibrating for one period, preventing the propagation of sound. The robustness of quadratic dispersion has been questioned by arguing that the anharmonic phonon–phonon interaction linearizes it. However, this implies a divergent bending rigidity in the long-wavelength regime. Here we show that rotational invariance protects the quadratic flexural dispersion against phonon–phonon interactions, and consequently, the bending stiffness is non-divergent irrespective of the temperature. By including non-perturbative anharmonic effects in our calculations, we find that sound propagation coexists with a quadratic dispersion. We also show that the temperature dependence of the height fluctuations of the membrane, known as ripples, is fully determined by thermal or quantum fluctuations, but without the anharmonic suppression of their amplitude previously assumed. These conclusions should hold for all two-dimensional materials.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
Source data are provided with this paper. All other data are available from the corresponding author upon reasonable request.
Code availability
The atomistic calculations of the SCHA theory are performed with the SSCHA code. This code is open source and can be downloaded from https://sscha.eu/. The SCHA calculations in the membrane model are performed with an in-house program adapting the distributed SCHA code, which is available from the corresponding author upon reasonable request.
References
Landau, L. D. & Lifshitz, E. M. Statistical Physics (Pergamon, 1980).
Mermin, N. D. Crystalline order in two dimensions. Phys. Rev. 176, 250 (1968).
Novoselov, K. S. et al. Electric field effect in atomically thin carbon films. Science 306, 666–669 (2004).
Novoselov, K. S. et al. Two-dimensional atomic crystals. Proc. Natl Acad. Sci. USA 102, 10451–10453 (2005).
Meyer, J. C. et al. The structure of suspended graphene sheets. Nature 446, 60–63 (2007).
Nelson, D. R. & Peliti, L. Fluctuations in membranes with crystalline and hexatic order. J. Phys. France 48, 1085–1092 (1987).
Fasolino, A., Los, J. H. & Katsnelson, M. I. Intrinsic ripples in graphene. Nat. Mater. 6, 858–861 (2007).
Gazit, D. Correlation between charge inhomogeneities and structure in graphene and other electronic crystalline membranes. Phys. Rev. B 80, 161406 (2009).
Gazit, D. Structure of physical crystalline membranes within the self-consistent screening approximation. Phys. Rev. E 80, 041117 (2009).
San-Jose, P., González, J. & Guinea, F. Electron-induced rippling in graphene. Phys. Rev. Lett. 106, 045502 (2011).
Bonilla, L. L. & Carpio, A. Ripples in a graphene membrane coupled to Glauber spins. J. Stat. Mech. 2012, P09015 (2012).
Guinea, F., Le Doussal, P. & Wiese, K.J. Collective excitations in a large-d model for graphene. Phys. Rev. B 89, 125428 (2014).
González, J. Rippling transition from electron-induced condensation of curvature field in graphene. Phys. Rev. B 90, 165402 (2014).
Ruiz-García, M., Bonilla, L. & Prados, A. Ripples in hexagonal lattices of atoms coupled to Glauber spins. J. Stat. Mech. 2015, P05015 (2015).
Gornyi, I. V., Kachorovskii, V. Y & Mirlin, A. D. Rippling and crumpling in disordered free-standing graphene. Phys. Rev. B 92, 155428 (2015).
Ruiz-García, M., Bonilla, L. L. & Prados, A. STM-driven transition from rippled to buckled graphene in a spin-membrane model. Phys. Rev. B 94, 205404 (2016).
Bonilla, L. L. & Ruiz-García, M. Critical radius and temperature for buckling in graphene. Phys. Rev. B 93, 115407 (2016).
Gornyi, I. V., Dmitriev, A. P., Mirlin, A. D. & Protopopov, I. V. Electron in the field of flexural vibrations of a membrane: quantum time, magnetic oscillations, and coherence breaking. J. Exp. Theor. Phys. 123, 322–347 (2016).
Ruiz-García, M., Bonilla, L. L. & Prados, A. Bifurcation analysis and phase diagram of a spin-string model with buckled states. Phys. Rev. E 96, 062147 (2017).
Cea, T., Ruiz-García, M., Bonilla, L. & Guinea, F. Numerical study of the rippling instability driven by electron-phonon coupling in graphene. Phys. Rev. B 101, 235428 (2020).
Silva-Guillén, J.A. & Guinea, F. Electron heating and mechanical properties of graphene. Phys. Rev. B 101, 060102 (2020).
Paulatto, L., Mauri, F. & Lazzeri, M. Anharmonic properties from a generalized third-order ab initio approach: theory and applications to graphite and graphene. Phys. Rev. B 87, 214303 (2013).
Bonini, N., Garg, J. & Marzari, N. Acoustic phonon lifetimes and thermal transport in free-standing and strained graphene. Nano Lett. 12, 2673–2678 (2012).
Wang, H. & Daw, M. S. Anharmonic renormalization of the dispersion of flexural modes in graphene using atomistic calculations. Phys. Rev. B 94, 155434 (2016).
Los, J. H., Katsnelson, M. I., Yazyev, O. V., Zakharchenko, K. V. & Fasolino, A. Scaling properties of flexible membranes from atomistic simulations: application to graphene. Phys. Rev. B 80, 121405 (2009).
Katsnelson, M. I. & Fasolino, A. Graphene as a prototype crystalline membrane. Acc. Chem. Res. 46, 97–105 (2013).
Zakharchenko, K. V., Katsnelson, M. I. & Fasolino, A. Finite temperature lattice properties of graphene beyond the quasiharmonic approximation. Phys. Rev. Lett. 102, 046808 (2009).
Mariani, E. & Von Oppen, F. Flexural phonons in free-standing graphene. Phys. Rev. Lett. 100, 076801 (2008).
Amorim, B. et al. Thermodynamics of quantum crystalline membranes. Phys. Rev. B 89, 224307 (2014).
De Andres, P. L., Guinea, F. & Katsnelson, M. I. Bending modes, anharmonic effects, and thermal expansion coefficient in single-layer and multilayer graphene. Phys. Rev. B 86, 144103 (2012).
Michel, K. H., Costamagna, S. & Peeters, F. M. Theory of anharmonic phonons in two-dimensional crystals. Phys. Rev. B 91, 134302 (2015).
Michel, K. H., Scuracchio, P. & Peeters, F. M. Sound waves and flexural mode dynamics in two-dimensional crystals. Phys. Rev. B 96, 094302 (2017).
Le Doussal, P. & Radzihovsky, L. Self-consistent theory of polymerized membranes. Phys. Rev. Lett. 69, 1209–1212 (1992).
Blees, M. K. et al. Graphene kirigami. Nature 524, 204–207 (2015).
Lindahl, N. et al. Determination of the bending rigidity of graphene via electrostatic actuation of buckled membranes. Nano Lett. 12, 3526–3531 (2012).
Al Taleb, A., Anemone, G., Farías, D. & Miranda, R. Acoustic surface phonons of graphene on Ni (111).Carbon 99, 416–422 (2016).
Al Taleb, A., Yu, H.K., Anemone, G., Farías, D. & Wodtke, A. M. Helium diffraction and acoustic phonons of graphene grown on copper foil. Carbon 95, 731–737 (2015).
Al Taleb, A., Anemone, G., Farías, D. & Miranda, R. Resolving localized phonon modes on graphene/Ir (111) by inelastic atom scattering. Carbon 133, 31–38 (2018).
Tømterud, M. et al. Temperature dependent bending rigidity of graphene. Preprint at https://doi.org/10.48550/arXiv.2210.17250 (2022).
Bianco, R., Errea, I., Paulatto, L., Calandra, M. & Mauri, F. Second-order structural phase transitions, free energy curvature, and temperature-dependent anharmonic phonons in the self-consistent harmonic approximation: theory and stochastic implementation. Phys. Rev. B 96, 014111 (2017).
Errea, I., Calandra, M. & Mauri, F. Anharmonic free energies and phonon dispersions from the stochastic self-consistent harmonic approximation: application to platinum and palladium hydrides. Phys. Rev. B 89, 064302 (2014).
Monacelli, L., Errea, I., Calandra, M. & Mauri, F. Pressure and stress tensor of complex anharmonic crystals within the stochastic self-consistent harmonic approximation. Phys. Rev. B 98, 024106 (2018).
Rowe, P., Csányi, G., Alfè, D. & Michaelides, A. Development of a machine learning potential for graphene. Phys. Rev. B 97, 054303 (2018).
Monacelli, L. & Mauri, F. Time-dependent self-consistent harmonic approximation: anharmonic nuclear quantum dynamics and time correlation functions. Phys. Rev. B 103, 104305 (2021).
Lihm, J.-M. & Park, C.-H. Gaussian time-dependent variational principle for the finite-temperature anharmonic lattice dynamics. Phys. Rev. Res. 3, L032017 (2021).
Wang, Z. K., Lim, H. S., Ng, S. C., Özyilmaz, B. & Kuok, M. H. Brillouin scattering study of low-frequency bulk acoustic phonons in multilayer graphene. Carbon 46, 2133–2136 (2008).
Landau, L. D. & Lifshits, E. M. Theory of Elasticity (Addison-Wesley, 1959).
Kownacki, J.-P. & Mouhanna, D. Crumpling transition and flat phase of polymerized phantom membranes. Phys. Rev. E 79, 040101 (2009).
Roldán, R., Fasolino, A., Zakharchenko, K. V. & Katsnelson, M. I. Suppression of anharmonicities in crystalline membranes by external strain. Phys. Rev. B 83, 174104 (2011).
Coquand, O. & Mouhanna, D. Flat phase of quantum polymerized membranes. Phys. Rev. E 94, 032125 (2016).
Hašík, J., Tosatti, E. & Martoňák, R. Quantum and classical ripples in graphene. Phys. Rev. B 97, 140301 (2018).
Wei, D. & Wang, F. Graphene: a partially ordered non-periodic solid. J. Chem. Phys. 141, 144701 (2014).
Cepellotti, A. et al. Phonon hydrodynamics in two-dimensional materials. Nat. Commun. 6, 6400 (2015).
Mahan, G. Many-Particle Physics. (Springer, 2000).
Dion, M., Rydberg, H., Schröder, E., Langreth, D. C. & Lundqvist, B. I. Van der Waals density functional for general geometries. Phys. Rev. Lett. 92, 246401 (2004).
Perdew, J. P., Burke, K. & Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865 (1996).
Vanderbilt, D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism. Phys. Rev. B 41, 7892 (1990).
Barone, V. et al. Role and effective treatment of dispersive forces in materials: polyethylene and graphite crystals as test cases. J. Comput. Chem. 30, 934–939 (2009).
Monkhorst, H. J. & Pack, J. D. Special points for Brillouin-zone integrations. Phys. Rev. B 13, 5188 (1976).
Ypma, T. J. Historical development of the Newton–Raphson method. SIAM Rev. 37, 531–551 (1995).
Acknowledgements
We would like to thank F. Guinea for useful conversations. Financial support was provided by the Spanish Ministry of Economy and Competitiveness (FIS2016-76617-P); the Spanish Ministry of Science and Innovation (grant nos. PID2019-105488GB-I00 and PID2022-142861NA-I00); the Department of Education, Universities and Research of the Basque Government and the University of the Basque Country (IT1707-22 and IT1527-22); and the European Commission under the Graphene Flagship, Core3, grant no. 881603. M.C. acknowledges support from ICSC—Centro Nazionale di Ricerca in HPC, Big Data and Quantum Computing, funded by the European Union under NextGenerationEU. U.A. is thankful to the Material Physics Center for a predoctoral fellowship. J.D. thanks the Department of Education of the Basque Government for a predoctoral fellowship (grant no. PRE-2020-1-0220). L.M. acknowledges funding support from the European Union under the Marie Curie Individual Fellowship THERMOH. Computer facilities were provided by the Donostia International Physics Center (DIPC).
Author information
Authors and Affiliations
Contributions
U.A. and J.D. contributed equally. U.A. performed the atomistic calculations, whereas U.A., J.D. and T.C. performed the calculations on the membrane and developed the theoretical adaptation of SCHA theory to the membrane. L.M., T.C., R.B. and I.E. developed the mathematical proof of the relation between Green’s function and free energy Hessian. I.E. and F.M. supervised the full project. The manuscript was written by U.A., J.D. and I.E., with input from all authors.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Peer review
Peer review information
Nature Physics thanks the anonymous reviewers for their contribution to the peer review of this work.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data
Extended Data Fig. 1 Negligible dynamic effects in the ZA mode frequencies.
Harmonic, and SCHA auxiliary and physical phonons (static and dynamic) calculated at 0 K (a) and 300 K (b) with the atomistic potential for the ZA mode.
Extended Data Fig. 2 Height-height correlation function as a function of the biaxial strain δa.
Impressively, the behavior for small q deviates from the q−4 law even for very small strains, for example δa = 10−5.
Extended Data Fig. 3 Bubble approximation in the static limit of the SCHA theory.
(a) Physical phonons in the static approach with the atomistic potential at 500 K including and neglecting \(\mathop{{{{\boldsymbol{\Phi }}}}}\limits^{(4)}\) in Eq. (11). (b) panel only includes the ZA modes and it is in logarithmic scale. The calculation is done in a 6 × 6 supercell.
Extended Data Fig. 4 Empirical potential benchmark I.
Harmonic phonon spectrum of graphene calculated with the machine learning empirical potential and ab initio. The calculations are done in a 6 × 6 supercell.
Extended Data Fig. 5 Empirical potential benchmark II.
Harmonic, and SCHA auxiliary and physical frequencies (static) using the DFT and machine learning (ML) forces. (a) panel shows the out-of-plane optical frequency at the Γ point and (b) panel the in-plane one.
Extended Data Fig. 6 Negative thermal expansion of graphene in the membrane model.
δa as a function of temperature in the membrane model.
Extended Data Fig. 7 Justification of the bubble and Lorentzian approximations in the calculation of spectral properties of the LA mode within the membrane model.
(a) Linewidth (full width at half maximum, FWHM) contribution of the term containing the fourth-order tensor of the LA mode calculated in the membrane model at 100 K using the harmonic and SCHA auxiliar phonons. The value of the smearing is in the legend. (b) Spectral function of the LA mode with momentum 0.01 Å−1 with and without considering the frequency dependence of the self energy.
Extended Data Fig. 8 Anharmonicity does not remove the quasiparticle picture of ZA modes.
Linewidth (full width half maximum) of ZA phonon mode divided by its frequency at 300K calculated within the membrane model. The ratio never gets bigger than 1.
Extended Data Fig. 9 Height-height correlation function showing a pure quantum behaviour.
Fourier transform of the height-height correlation function at 0 K in the membrane model evaluated at different levels of approximation: harmonic (black dots), anharmonic rotationally invariant (RI) result (green filled dots) and anharmonic no rotationally invariant (No RI) result (green empty dots). The dashed lines correspond to the linear fitting in each case.
Extended Data Fig. 10 Height-height correlation function showing a pure classical behaviour.
Fourier transform of the height-height correlation function at 300 K in the membrane model evaluated at different levels of approximation: harmonic (black dots), anharmonic RI result (green filled dots) and anharmonic No RI result (green empty dots). The dashed lines correspond to the linear fitting in each case.
Source data
Source Data Fig. 1
Numerical data. Comments for each panel are indicated with #.
Source Data Fig. 2
Numerical data. Comments for each panel are indicated with #.
Source Data Fig. 3
Numerical data. Comments for each panel are indicated with #.
Source Data Fig. 4
Numerical data. Comments for each panel are indicated with #.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Aseginolaza, U., Diego, J., Cea, T. et al. Bending rigidity, sound propagation and ripples in flat graphene. Nat. Phys. (2024). https://doi.org/10.1038/s41567-024-02441-z
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41567-024-02441-z